The perimeter of a triangle is the distance around the triangle. In other words, the perimeter is the sum of the lengths of the sides of the triangle. You will learn different ways to find the perimeter based on the different types of triangles.
Perimeter of a triangle formula
Start with a scalene triangle and label the sides as shown below.
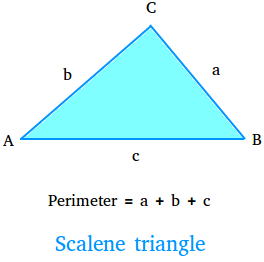
Let a, b, and c be the lengths of the sides of the triangle ABC and let P be the perimeter. Here is how to find the perimeter (P) or distance around the outside of the triangle.
P = a + b + c
Therefore, the formula to use to find the perimeter of a scalene triangle is P = a + b + c.
The perimeter is expressed in units.
- If a, b, c are measured in feet, then the perimeter is measured in feet or ft.
- If a, b, and c are measured in yard, then the perimeter is measured in yards or yd.
- If a, b, and are measured in inches, then the perimeter is measured in inches or inch.
Examples showing how to find the perimeter of a scalene triangle
Example #1
Find the perimeter of a triangle when a = 3 cm, b = 5 cm, and c = 7 cm
P = 3 + 5 + 7 = 15 cm
Example #2
Find the perimeter of a triangle when a = 5 feet, b = 8 feet, and c = 12 feet
P = 5 + 8 + 12 = 25 feet
Example #3
Find the perimeter when a = 3/2 cm, b = 5/2 cm, and c = 6/2 cm
P = 3/2 + 5/2 + 6/2 = (3 + 5 + 6)/2 = 14/2 = 7 cm
Perimeter of an equilateral triangle
Since the side lengths of an equilateral triangle are all equal, you can just multiply the length of one side by 3 to get the perimeter.
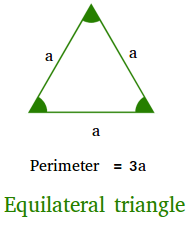
Let a be the length of one side of an equilateral triangle and let P be the perimeter. Here is how to find the perimeter (P) or distance around the outside of an equilateral triangle.
P = a + a + a = 3 × a
Therefore, the formula to use to find the perimeter of an equilateral triangle is P = 3 × a.
Examples showing how to find the perimeter of an equilateral triangle
Example #4
Find the perimeter of an equilateral triangle if the length of one side is 6
P = 3 × a = 3 × 6 = 18
Example #5
Find the perimeter of an equilateral triangle if the length of one side is 1/3 meter.
P = 3 × a = 3 × 1/3
P = 3/1 × 1/3 = (3 × 1)/(1 × 3) = 3/3 = 1
The perimeter is 1 meter when the length of one side is one-third or 1/3.
Perimeter of an isosceles triangle
An isosceles triangle has two sides that are equal. Let a and a be the lengths of the two sides that are equal.
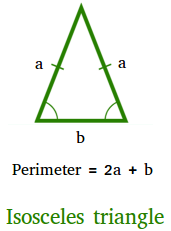
Let b be the length of the third side.
Let P be the perimeter. Here is how to find the perimeter (P) or distance around the outside of an isosceles triangle.
P = a + a + b = 2 × a + b
Therefore, the formula to use to find the perimeter of an isosceles triangle is P = 2 × a + b.
Examples showing how to find the perimeter of an isosceles triangle
Example #6
Find the perimeter of an isosceles triangle if the length of the two equal sides is 10 inches and the length of the third side is 15 inches.
P = 2 × a + b = 2 × 10 + 15
P = 20 + 15 = 35 inches
Example #7
Find the perimeter of an isosceles triangle if the length of the two equal sides is 1.5 cm and the length of the third side is 2 cm.
P = 2 × a + b = 2 × (1.5) + 2
P = 3 + 2 = 5 cm
Perimeter of a right triangle using the Pythagorean theorem
Suppose the lengths of the legs of a right-angled triangle are known, but the length of the hypotenuse is not known. In this case, you need to look for the length of the hypotenuse before looking for the perimeter.
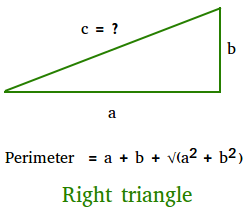
Let a and b be the lengths of the legs and let c be the length of the hypotenuse.
c2 = a2 + b2
c = √(a2 + b2)
Perimeter = a + b + √(a2 + b2)
Examples showing how to find the perimeter of a right triangle using the Pythagorean theorem
Example #8
Find the perimeter of a right triangle if the lengths of the legs are 6 and 8.
Perimeter = a + b + √(a2 + b2)
Perimeter = 6 + 8 + √(62 + 82)
Perimeter = 14 + √(36 + 64)
Perimeter = 14 + √(100) = 14 + 10 = 24
Example #9
Find the perimeter of a right triangle if the lengths of the legs are 7 and 24.
Perimeter = a + b + √(a2 + b2)
Perimeter = 7 + 24 + √(72 + 242)
Perimeter = 31+ √(49 + 576)
Perimeter = 31 + √(625) = 31 + 25 = 56
Perimeter of an SAS triangle
A triangle when two sides and the angle between these two sides are known is an SAS triangle or a side-angle-side triangle. Since you already know two sides of the triangle, all you need to do is to use the Law of Cosines to find the third side.
Once you find the third side, add the three sides to find the perimeter of the triangle.
How to find the length of a side of the triangle when the perimeter and two sides are given
Example #10
If P = 30 cm and a = 5 and b = 7, what is c?
Using the formula P = a + b + c, replace everything you know or everything given to you into the formula.
Things that are given are P = 30, a = 8, and b = 10
Replacing them into the formula gives:
30 = 8 + 10 + c
30 = 18 + c
You end up with an addition equation that you can solve to get c.
However, some mental math should provide you with an answer too.
Replace c by a question mark (?) and say to yourself the following:
18 + ? = 30 or what can I add to 18 to get 30 ? 18 + 12 = 30, so c = 12
Example #11
If P = 20 cm, b = 7 and c = 8, what is a?
Using the formula P = a + b + c, replace everything you know or everything given to you into the formula.
Things that are given are P = 20, b = 7, and c = 8
Replacing them into the formula gives:
20 = a + 7 + 8
20 = a + 15
What can I add to 15 to get 20? The answer is 5, so a = 5
Leave a Reply