The slope intercept form of a linear equation is y = mx + b, where m is the slope and b is the y-intercept. The graph of a linear equation is a line and y = mx + b is one of the most common forms to write the equation of a line.
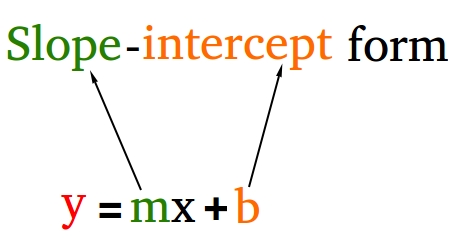
How to derive the slope intercept form of a linear equation
Consider an arbitrary point (x,y) on the line and a point (0,b) on the y-axis. Then, you can use the slope formula to derive the slope-intercept form.
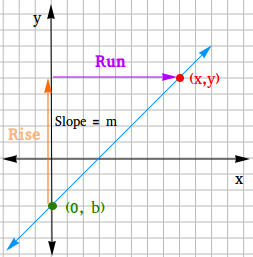
Slope = Rise / Run = (y1 – y2) / (x1 – x2)
Using (x1,y1) = (x,y) and (x2,y2) = (0,b), compute the slope m.
m = (y – b) / (x – 0)
m = (y – b) / x
Multiply both sides of the equation by x
(x)m = y – b
mx = y – b
Add b to both sides of the equation
mx + b = y – b + b
y = mx + b
Slope intercept formula
The slope intercept formula is the equation y = mx + b
m is the slope of the line
b is the y-intercept or the point on the y-axis
x and y are the x and y coordinates
Examples showing how to find the slope-intercept form of a straight line
The goal of these exercises is to write the equation of a straight line in slope-intercept form (y = mx + b) by considering the following cases:
- The slope and the y-intercept are given (example #1)
- The slope and a point on the line are given (example #2)
- Two points on the line are given (example #3)
Example #1
If the slope of a line is m = 2 and the y-intercept is b = 5, write the slope intercept form of the line.
The equation is y = 2x + 5.
Example #2
If the slope of a line is m = 5 and (1, 6) is a point on the line, find the slope intercept form of the line.
This time we have m, but b is missing, so we have to find b.
Since m = 5, y = mx + b becomes y = 5x + b.
Now, use (1, 6) to get b.
Since x = 1 and y = 6, you can replace them into the equation.
Substituting 1 for x and 6 for y gives 6 = 5×1 + b.
6 = 5×1 + b is just a linear equation that you can solve to get b.
6 = 5×1 + b
6 = 5 + b
Subtract 5 from both sides.
6 − 5 = 5 − 5 + b
1 = 0 + b
1 = b
Now since we have b, y = 5x + 1
Example #3
Suppose (2, 3) and (4, 9) are two points on a line. Find the slope intercept form of the line.
This time both m and b are missing, so the first thing to do is to get m and then use m and a point, either (2, 3) or (4, 9) to get b.
Let x1 = 4, y1 = 9 and x2 = 2, y2 = 3
m = (y1 − y2) / (x1 − x2) = (9 − 3) / (4 − 2 ) = 6 / 2 = 3
Now we can use the value for m and one point to get b as already done in example #2.
Although you have two points, It does not matter which point you choose. Since both points are on the line, they will yield similar results.
Choosing (2, 3), x = 2 and y = 3
Substituting 2 for x, 3 for y, and 3 for m into the equation y = mx + b we get:
3 = 3 × 2 + b
3 = 6 + b
Subtract 6 from both sides
3 − 6 = 6 − 6 + b
-3 = 0 + b
-3 = b
Now we have b = -3 and m = 3, y = 3x + -3
Slope intercept form special cases
The y-intercept b is equal to zero
In this case, y = mx and the line always goes through the origin of the coordinate system.
The slope of the line is equal to zero
In this case, y = (0)x + b = b and when you graph the line, it will be a horizontal line as it crosses the y-intercept.
The slope of the line is undefined
In this case, x is equal to the x-coordinate of any point on the line or any other number that is given to you in an exercise. When you graph the line, it will be a vertical line as it crosses the x-intercept.
Leave a Reply