Now, we show you a math trick that you can use to square a number easily and fast. Take a close look at the figure below! It shows how you can use a cool math trick to square a two-digit number much faster than the traditional way of doing it.
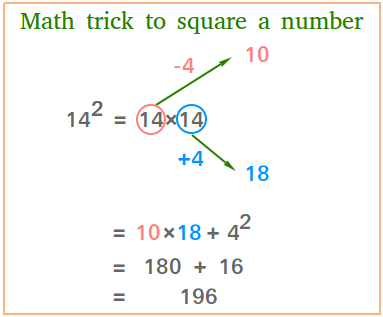
The goal is to rewrite one of the numbers being multiplied so that it will have 1 or more zeros.
At this point, there may be one thing that you may not quite understand. Why did we add 42 to 10 × 18? Keep reading as we show you the math behind it!
10 × 18 = (14 – 4)(14 + 4)
10 × 18 = 14 × 14 + 14 × 4 – 14 × 4 – 42
10 × 18 = 14 × 14 + 56 – 56 – 42
10 × 18 = 14 × 14 – 42
Now, all we have to do is to add 42 to both sides of 10 × 18 = 14 × 14 – 42
10 × 18 + 42 = 14 × 14 – 42 + 42
10 × 18 + 42 = 14 × 14 (Here we go!)
In general,
Let X and c be a natural number. If X + c or X – c gives a number that has 1 or more zeros, then you can multiply X by X easily using the equation X2 = (X + c)(X – c) + c2
Example #7
172 = (17 – 7)(17 + 7) + 72
172 = (10)(24) + 49
172 = 240 + 49
172 = 289
Leave a Reply