The volume of a cube is how much space the cube occupies. Given the length of one side, call it a, the volume of any cube can be found by using the following formula:
Volume of a cube formula
Volume of any cube = a3 = a × a × a
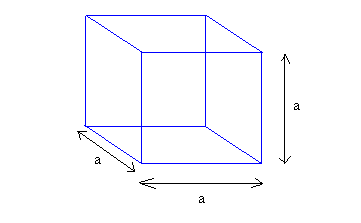
If a is the edge length of the cube, the volume of the cube is the cube of the edge length or the edge length raised to the third power.
The volume is expressed in cubic units.
- If a is measured in meters, then the volume is measured in cubic meters or m3.
- If a is measured in centimeters, then the volume is measured in cubic centimeters or cm3.
- If a is measured in inches, then the volume is measured in cubic inches or in.3
Examples showing how to find the volume of a cube
Example #1:
Find the volume of a cube if the length of one side is 2 cm
Since a is equal to 2, volume of the cube = a3 = 23
Volume of the cube = 2 × 2 × 2
Volume of the cube = 4 × 2
Volume of the cube = 8 cm3
Example #2:
Find the volume if the length of one side is 3 cm
Since a is equal to 3, volume of the cube = a3 = 33
Volume of the cube = 3 × 3 × 3
Volume of the cube= 9 × 3
Volume of the cube = 27 cm3
Example #3:
Find the volume of a cube if the length of one side is 3/2 cm
Volume of the cube = (3/2)3
Volume of the cube = 3/2 × 3/2 × 3/2
Volume of the cube = (3 × 3 × 3)/(2 × 2 × 2)
Volume of the cube = 27/8 cm3
Volume of the cube = 3.375 cm3
Real-life examples showing how to find the volume of a cube
Example #4:
Each edge of a Rubik’s cube has a length of about 5.7 cm. What is the volume of the Rubik’s cube?
Volume of the Rubik’s cube = (5.7)3
Volume of the Rubik’s cube = 5.7 × 5.7 × 5.7
Volume of the Rubik’s cube = (32.49) × 5.7 = 185.193
Volume of the Rubik’s cube = 185.193 cm3
Example #5:
What is the volume of an ice cube that is (3/4)” by (3/4)” by (3/4)” ?
Volume of the ice cube = (3/4)3
Volume of the ice cube = (3/4) × (3/4) × (3/4)
Volume of the ice cube = (3 × 3 × 3)/(4 × 4 × 4)
Volume of the ice cube = (27)/(64) in.3
Volume of the ice cube = 0.4218.75 in.3
Using the diagonal of a cube to find the volume of the cube
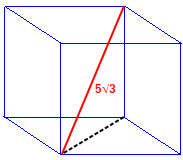
Example #6:
The length of the diagonal of a cube shown in red above is 5√3. Find the volume of the cube.
The diagonal of the cube is the hypotenuse of a right triangle formed by the edge of the cube and the diagonal of a face. Therefore, you can use the Pythagorean theorem to find the length of the side of the cube.
You will need to do this in two steps!
Step #1
Find the length of the diagonal of a face shown in black.
Let x be the length of the diagonal of a face and let a be the edge length.
x2 = a2 + a2
x2 = 2a2
x = √(2a2)
x = (√2)a
Step #2
Find the edge length using of the length of the diagonal of the cube and the length of the diagonal of a face.
Use the Pythagorean theorem again!
(5√3)2 = [(√2)a]2 + a2
25(3) = 2(a)2 + a2
75 = 3a2
25 = a2
a = 5
Volume of the cube = 53 = (5)(5)(5) = 125
Leave a Reply